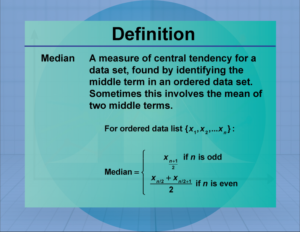
The median definition is an important concept in math and statistics. The median is the middle number in a set of numbers when they are arranged in order. If there are two middle numbers, we find the average of those two to get the median.
Knowing how to find the median can help us understand data better. For example, if we have the ages of five friends—8, 10, 12, 14, and 16—the median is 12 because it is right in the middle. This helps us see what the “middle” age is in this group.
What is the Median Definition
The median definition is a simple idea in math. It is the middle number in a list of numbers when they are arranged from smallest to largest. For example, if we have the numbers 1, 3, 5, and 7, we first organize them. The median is the number that sits in the middle. If there is an odd number of numbers, like in the list 2, 4, 6, 8, and 10, the middle number is 6.
Understanding this concept is very helpful. It allows us to find a value that represents a set of data. When we have a group of scores or ages, knowing the median helps us see what is typical. This is important in many areas, like sports, school, and even in everyday life.
Calculating the median is easy once you know how. Begin by putting the numbers in order. Next, count how many numbers there are. If there are two middle numbers, you take their average. This way, the median gives a fair view of the data.
How to Find the Median: A Step-by-Step Guide
Finding the median is a simple process. First, you need to arrange the numbers in order from smallest to largest. If you have the numbers 5, 2, 9, and 1, you will sort them to be 1, 2, 5, and 9. After that, count how many numbers are in the list.
If the total number of numbers is odd, the median is the middle one. For instance, in the list 3, 7, 8, the middle number is 7. However, if there is an even number, like 4, 6, 8, and 10, you will find the two middle numbers, which are 6 and 8. The average of these two is 7, which is the median.
Using this method helps you find the median in any group of numbers. It can be ages, scores, or other data. With practice, you will become fast at finding the median.
Why is the Median Important in Math
The median definition is very important in math and statistics. It helps us understand data clearly. Sometimes, when we look at numbers, there can be some very high or very low values. These can make the average misleading. The median gives a clearer picture because it focuses on the middle.
For example, imagine a classroom where most students score between 70 and 80 on a test, but one student scores 100. The average score may seem high because of that one score. However, the median score would be around 75, showing what most students scored. This helps teachers understand the overall class performance.
Additionally, the median is often used in research and surveys. It allows researchers to summarize data effectively. By focusing on the median, they can present a clearer view of the results, helping them make better decisions.
Median vs. Mean: Understanding the Difference
When discussing the median definition, it’s common to compare it with the mean. The mean is calculated by adding all the numbers together and then dividing by how many numbers there are. The median, on the other hand, is just the middle number.
This difference is important because they can tell us different stories about the data. For instance, if you have the numbers 2, 4, 6, and 100, the mean will be much higher because of the 100. However, the median will be 5, which is more reflective of the majority of the numbers.
Knowing the difference between median and mean helps us understand data better. It allows us to choose the best measure to describe a situation. Sometimes, the median is more accurate than the mean, especially when there are extreme values.
Real-Life Examples of Median Definition
The median definition appears in many real-life situations. For example, when looking at test scores in a class, the median helps show how students performed. If most students score between 60 and 80, the median score will represent the typical score better than the average.
Another great example is in sports. Coaches often look at players’ statistics, such as points scored in a game. The median can show them what a typical score looks like, which helps them make decisions. This is especially useful when some players have very high or very low scores.
In shopping, the median price of products can help buyers understand what is reasonable. If you want to buy a new phone, knowing the median price helps you see what most phones cost, guiding your choices wisely.
How to Calculate the Median in Different Sets
Calculating the median is the same for different sets of numbers. Whether you are looking at ages, test scores, or anything else, the steps stay the same. Start by sorting the numbers from smallest to largest. This is the first step in finding the median.
Next, count how many numbers are in the list. If there are an odd number, the median is simply the middle number. For example, if the list is 1, 2, 3, 4, and 5, the median is 3. If there is an even number, like in the set 2, 4, 6, and 8, you find the two middle numbers, which are 4 and 6, then average them to get 5.
These steps work for any set of numbers you encounter. With practice, you can quickly calculate the median, making it a useful skill in math and everyday life.
The Role of Median in Statistics
The median definition plays a big role in statistics. It is a crucial measure that helps us summarize data without being affected by extreme values. For example, in a survey about family incomes, the median helps show what a typical family earns. If one family earns a lot more, it won’t change the median much.
Researchers rely on the median to understand trends and patterns in data. By using the median, they can present information clearly, making it easier for everyone to understand. This is why the median is often included in reports and studies.
Statisticians know that the median provides a reliable measure of central tendency. This means it helps describe where most values lie in a dataset. The role of the median is vital in many fields, including economics, social science, and health.
Median in Graphs: What Does It Show?
When we visualize data using graphs, the median definition helps us understand information quickly. In a box plot, for example, the median is shown as a line inside the box. This indicates the middle value of the dataset, making it easy to see where most numbers fall.
Graphs can also show how numbers are spread out around the median. If most numbers are close to the median, the data is considered tightly grouped. But if there are many numbers far away from the median, it indicates a wider spread. Understanding this can help us interpret data effectively.
Using graphs with median values allows us to see trends over time. For example, a line graph can show how a student’s grades change. By looking at the median grade over several tests, we can see if the student is improving or struggling.
Common Mistakes When Finding the Median
Finding the median definition might seem easy, but there are common mistakes to watch for. One frequent error is not arranging the numbers in order. If the numbers are mixed up, the median will be incorrect. Always start by sorting them!
Another mistake occurs when counting the numbers. If you forget how many numbers are in the list, you might pick the wrong median. It is essential to keep track of the total number of numbers before finding the median.
Also, remember that when there is an even number of values, you need to average the two middle numbers. Forgetting this can lead to an incorrect median. By being careful and following the steps, you can avoid these mistakes and accurately find the median every time.
Fun Activities to Practice Median Definition
Learning about the median definition can be fun! One activity is to ask friends or family for their ages. Write down the ages, sort them, and find the median. This is a great way to practice while also learning about the people around you.
Another fun activity is to track your favorite sports team’s scores. Record the scores from several games, arrange them in order, and find the median score. This not only helps you learn but also gives you insight into how well your team is performing.
You could also create a game where you challenge friends to find the median of various sets of numbers. Set a timer and see who can find the median the fastest. Making learning interactive helps everyone remember the concept better.

Using Median in Everyday Life
Understanding the median definition can help in daily life. When shopping, knowing the median price of items helps you find good deals. If you are looking for clothes, checking the median price tells you what is normal, helping you avoid overpaying.
In school, teachers use the median to evaluate student performance. By looking at the median grades, they can see how the class is doing as a whole. This can help them decide if they need to review certain topics or provide extra help to students who are struggling.
In conversations, you might hear about the median age in a community. This information helps people understand the population better. By knowing the median age, local leaders can plan activities and services that meet the needs of the community.
Conclusion
In conclusion, the median definition is an important idea in math that helps us find the middle number in a group. Knowing how to calculate the median is useful in many situations, like school, shopping, and even sports. When we understand the median, we can make better choices based on the data we have. It shows us what is typical and helps us avoid getting confused by very high or very low numbers.
Learning about the median can be fun and easy! By practicing with different sets of numbers and using real-life examples, you can become a median master. Remember, the median helps us see the true picture of our data, making it a valuable tool in math and everyday life. So next time you have a list of numbers, try finding the median and see what it tells you.


